Introduction
I love to look for physical interpretations of various constants. Sometimes it is impossible to come up with an interpretation, but such is not the case for the ballistic coefficient. This morning I read a very solid piece of technical work on the ballistic coefficient that has another interpretation that I have not seen before (Source). Here is a statement they made that I will support with a quick derivation.
To a rough approximation, the BC [ballistic coefficient] can be estimated as the fraction of 1000 yards over which a projectile loses half of its initial kinetic energy. In other words, a bullet with a BC of 0.300 should lose roughly half of its initial kinetic energy at a range of 300 yards.
This statement is very interesting in a number of ways:
- Can I derive it using the expressions from Pejsa's book "Modern Practical Ballistics"?
- What do they mean by "rough approximation"?
- Does this approximation work with bullets that have a ballistic coefficient greater than 1?
I will address all three questions in this post. Let's dig in ...
Background
The ballistic coefficient is a very old school concept -- the ballistic coefficient is the ratio of a projectile's deceleration due to drag compared to the deceleration due to drag for a reference projectile of the same shape. Modern projectile simulation software would use different approaches (see McCoy). For my purposes here, we will define the ballistic coefficient C as shown in Equation 1.
| Eq. 1 |  |
where
- aRef is the deceleration of a reference projectile.
- aProjectile is the deceleration of our projectile under test.
Analysis
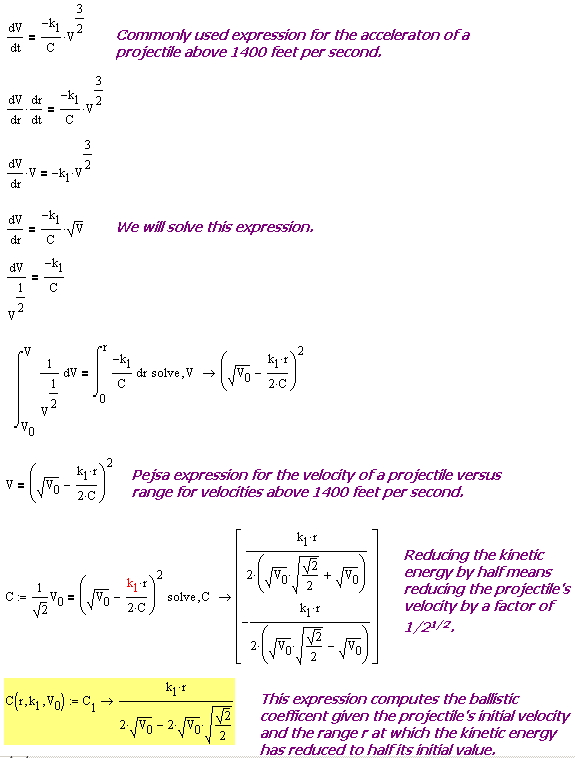
Figure 1 shows my derivation of an expression for the ballistic coefficient as a function of projectile velocity and range. The derivation presumes that reducing the kinetic energy by a factor of 2 means a velocity reduction by the 
Figure 1: Derivation of an Expression for the Ballistic Coefficient.
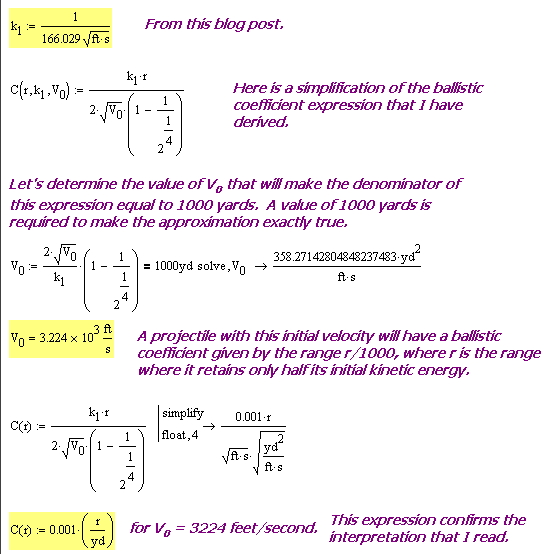
Figure 2 complete my confirmation of the approximation. The approximation is exactly true for projectiles with an initial velocity of 3224 feet per second. It is approximately true for initial velocities near 3224 feet per second, which is common for high-velocity rifle projectiles.
Figure 2: Verification of the Approximation.
Conclusion
Let's review my answers to the questions I presented in my Introduction.
- Can I derive it using the expressions from Pejsa's book "Modern Practical Ballistics"?
See Figure 2.
- What do they mean by "rough approximation"?
It is only exactly true for projectiles with an initial velocity of 3224 feet per second. It is only roughly true for initial projectile velocities near 3224 feet per second.
- Does this approximation work with bullets that have a ballistic coefficient greater than 1?
It is not too bad for a battleship projectile. Using the approximation and the range table here, I get that the 16 inch ballistic coefficient is ~13 (actual value of ~15). Not too bad considering the initial velocity is 2577 feet per second instead of 3224 feet per second.
On a distantly related topic are you able to estimate the drag coefficient of a race horse and jockey, head and side on? Seems to be a missing topic.
Funny! I am sure we could estimate it. I did estimate the drag coefficient for Felix Baumgartner on his parachute jump (post). I guess you could do similar calculations for almost anything.
mathscinotes
Thanks - I have asked all over without success - are you going to try it out?.
Coincidentally, my late father was in the UK Royal Navy and developed the early computer guided ship's gunnery systems. They used to test fire at drone targets 20 miles out into the English Channel from HMS Fraser in Portsmouth, UK.
I don 't have any data on the forces on horses and jockeys, so I would not know where to even start.
As far as your father's background, I am very interested in how early fire control systems work. My interest is driven by my interests in all things analog. I am always amazed at how well those systems performed.
mathscinotes
Always start with a first step. Perhaps the geometry can be scaled form photographs and Muybridge step photography used. Unlike F1 cars much of the horse driving mechanism - the legs- are exposed and accelerate/ decelerate rapidly. It is a fascinating problem.
My late father served through WW2 and I understand USA Navy then used a lot of gunnery and fire control methods from the UK Royal Navy. The WW2 analogue methods are well described at:
http://www.hmshood.com/ship/fire_control.htm
Battleship HMS Hood was sunk by the Bismark in 1941 losing all but 3 of its 1418 crew. A tremendous blow to UK moral at the time.
My father moved the analogue systems onto I presume analogue computers in the late 1950s
Robert
How is the ballistic coefficient related to armor penetration? Please let me know.
I don't believe they are related at all. Armor penetration usually requires projectiles that are not optimal aerodynamically. For an example, see the 16-inch projectiles I show on this page . Notice how a ballistic cover is placed on the armor-piercing portion of the shell to make it more aerodynamically efficient.
The question of armor penetration is part of the subject of terminal ballistics. I have not done much work in that area.
mathscinotes
Pingback: Ballistic Coefficient Rule of Thumb Example | Math Encounters Blog
Where do you get the 166.029 for calculating the K1 value? The equation is K1 = 1 / (166.029 (ft*s)) but I'm not too sure how you got that number.
Thanks!